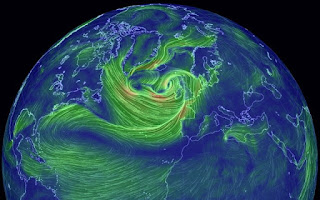
The hairy ball theorem and why there is no wind (somewhere) on Earth
< change language
What if I told you that right now there is a place on Earth where there is no wind blowing to the sides? None at all! How can I know that? All we need is what is usually called the Hairy Ball Theorem.
In less rigorous contexts, one can phrase the Hairy Ball Theorem as such:
Theorem (Hairy Ball I): if you have a hairy ball, regardless of the way you comb its hair there will always be a spot where the hair points right up.
In this particular image, the hair is pointing up both on the top and on the bottom.
More formally, the Hairy Ball Theorem can be formulated like so:
Theorem (Hairy Ball II): every continuous vector field over $S^2$ has at least a point where the tangential component is $0$.
From this theorem it is actually quite easy to establish our interesting fact!
If we think of the wind at the Earth's surface as a continuous vector field, the Hairy Ball Theorem says that there must be a point where the wind isn't blowing to the sides!
Now all that is left is proving the theorem! How can one do that? I will resort to algebraic topology because it enables a proof that I think it quite intuitive.
For this proof one needs to know what is the degree of a continuous mapping from $S^n$ to $S^n$. Throwing all rigour off the window, the degree of a continuous mapping $f: S^n \to S^n$ can be seen as a measurement of how many times $f(S^n)$ will wrap around $S^n$.
Assume we are working with $S^1$ (in $S^1$ the "degree" is usually called "winding number"). Imagine two circles side by side and, as you travel around the left circle, on top of some point $P$, you mark the image $f(P)$ on the right circle. The degree of $f$ will be the number of turns you did on the right circle after completing one turn on the left one.
The animation above shows what would happen if $f(P) = P$, i.e. $f = id$. When the white dot completes one turn the red dot does the same, hence $deg(f) = 1$.
As another example, write $P = (\cos(\theta), \sin(\theta))$ and let $f_n(P) = (\cos(n\theta), \sin(n\theta))$. Then for $f_3$ we have:
$deg(f_3) = 3$, because the red dot completes three turns when the white dot completes one.
For $f_{-1}$ we have $deg(f_{-1}) = -1$, as you can see below:
When the white dot completes one turn in the positive direction, the red dot completes one turn in the negative direction, hence the $-1$.
Of course we can think of other continuous mappings, for example $f(P) = -P$, which sends a point to its antipodal point. In that case, $deg(f) = 1$:
With the notion of degree of a continuous mapping in mind, we state that the identity function $id: S^2 \to S^2$ has degree $1$ and the antipodal function $f: S^2 \to S^2$, $f: P \mapsto -P$ has degree $-1$ (notice that in $S^1$ the antipodal map had degree $1$!). We now proceed to prove the Hairy Ball Theorem by contradiction.
We have that the degree of a continuous map $f$ is invariant under homotopy, i.e. if $f$ is continuously deformed, its degree remains unchanged. On the other hand, if there is a nonzero tangent vector field on $S^2$, for each point $P$ we set $v_P$ as that nonzero vector; with it, we define the homotopy $H: S^2 \times [0, \pi] \to S^2$ such that $H(P, \theta)$ is the point that is $\theta$ radians away from $P$ on the arc that goes from $P$ to $-P$ with the direction of $v_P$.
It should be clear that $H$ is continuous, $H(P, 0) = P$ and $H(P, \pi) = -P$, making $H$ an homotopy between $id$ and $-id$, an impossibility! (impossible because they are functions with different degrees). Having reached this contradiction, we conclude that $v_P$ must have not been well defined and therefore there was a point $P$ where the tangential component of the vector field was $0$!
What did you think of this? Who would say that mathematics could produce such bizarre results?
In less rigorous contexts, one can phrase the Hairy Ball Theorem as such:
Theorem (Hairy Ball I): if you have a hairy ball, regardless of the way you comb its hair there will always be a spot where the hair points right up.
In this particular image, the hair is pointing up both on the top and on the bottom.
More formally, the Hairy Ball Theorem can be formulated like so:
Theorem (Hairy Ball II): every continuous vector field over $S^2$ has at least a point where the tangential component is $0$.
From this theorem it is actually quite easy to establish our interesting fact!
If we think of the wind at the Earth's surface as a continuous vector field, the Hairy Ball Theorem says that there must be a point where the wind isn't blowing to the sides!
Now all that is left is proving the theorem! How can one do that? I will resort to algebraic topology because it enables a proof that I think it quite intuitive.
For this proof one needs to know what is the degree of a continuous mapping from $S^n$ to $S^n$. Throwing all rigour off the window, the degree of a continuous mapping $f: S^n \to S^n$ can be seen as a measurement of how many times $f(S^n)$ will wrap around $S^n$.
Assume we are working with $S^1$ (in $S^1$ the "degree" is usually called "winding number"). Imagine two circles side by side and, as you travel around the left circle, on top of some point $P$, you mark the image $f(P)$ on the right circle. The degree of $f$ will be the number of turns you did on the right circle after completing one turn on the left one.
The animation above shows what would happen if $f(P) = P$, i.e. $f = id$. When the white dot completes one turn the red dot does the same, hence $deg(f) = 1$.
As another example, write $P = (\cos(\theta), \sin(\theta))$ and let $f_n(P) = (\cos(n\theta), \sin(n\theta))$. Then for $f_3$ we have:
$deg(f_3) = 3$, because the red dot completes three turns when the white dot completes one.
For $f_{-1}$ we have $deg(f_{-1}) = -1$, as you can see below:
When the white dot completes one turn in the positive direction, the red dot completes one turn in the negative direction, hence the $-1$.
Of course we can think of other continuous mappings, for example $f(P) = -P$, which sends a point to its antipodal point. In that case, $deg(f) = 1$:
With the notion of degree of a continuous mapping in mind, we state that the identity function $id: S^2 \to S^2$ has degree $1$ and the antipodal function $f: S^2 \to S^2$, $f: P \mapsto -P$ has degree $-1$ (notice that in $S^1$ the antipodal map had degree $1$!). We now proceed to prove the Hairy Ball Theorem by contradiction.
We have that the degree of a continuous map $f$ is invariant under homotopy, i.e. if $f$ is continuously deformed, its degree remains unchanged. On the other hand, if there is a nonzero tangent vector field on $S^2$, for each point $P$ we set $v_P$ as that nonzero vector; with it, we define the homotopy $H: S^2 \times [0, \pi] \to S^2$ such that $H(P, \theta)$ is the point that is $\theta$ radians away from $P$ on the arc that goes from $P$ to $-P$ with the direction of $v_P$.
It should be clear that $H$ is continuous, $H(P, 0) = P$ and $H(P, \pi) = -P$, making $H$ an homotopy between $id$ and $-id$, an impossibility! (impossible because they are functions with different degrees). Having reached this contradiction, we conclude that $v_P$ must have not been well defined and therefore there was a point $P$ where the tangential component of the vector field was $0$!
What did you think of this? Who would say that mathematics could produce such bizarre results?
The animations were made by me with code that can be found here.
E se eu dissesse que neste exato instante há um sítio na Terra onde não há nenhum vento a soprar para os lados? Mesmo nenhum! Como é que eu posso afirmar isto? Na verdade, não preciso de mais nada para além do Teorema da Bola Cabeluda.
Em contextos menos rigorosos este teorema aparece escrito da seguinte forma:
Teorema (Bola Cabeluda I): se eu tiver uma bola cabeluda, independentemente do modo como a penteie haverá sempre um ponto onde os cabelos estão espetados no ar.
Em particular, nesta imagem em cima o cabelo está espetado tanto no topo como na parte de baixo da bola.
De um modo mais formal, o Teorema da Bola Cabeluda pode ser formulado como se segue:
Teorema (Bola Cabeluda II): qualquer campo vetorial contínuo sobre $S^2$ tem pelo menos um ponto onde a componente tangencial é $0$.
Com este teorema torna-se bastante fácil provar o nosso resultado!
Se pensarmos no vento sobre a superfície da Terra como um campo vetorial contínuo, o Teorema da Bola Cabeluda diz que tem de haver um ponto onde o vento não está a soprar para os lados!
Tudo o que nos falta agora é provar o teorema! Como podemos fazer isso? Vou usar uma prova da topologia algébrica porque eu acho que é uma prova relativamente intuitiva.
Para esta prova é preciso saber o que é o grau de uma função contínua de $S^n$ para $S^n$. Sem qualquer rigor, o grau de uma função contínua $f: S^n \to S^n$ pode ser visto como uma medida de quantas vezes $f(S^n)$ vai ser enrolado à volta de $S^n$.
Assumamos que estamos a trabalhar em $S^1$ (em $S^1$, o "grau" que se diz degree em inglês também tem o nome particular de winding number). Imaginem-se dois círculos lado a lado e, à medida que viajamos sobre o círculo da esquerda, em cima de um ponto $P$, vamos marcando no círculo da direita a imagem $f(P)$. O grau de $f$ vai ser o número de voltas completas que demos no círculo da direita quando acabarmos uma volta em cima do círculo da esquerda.
A animação em cima concretiza esta minha descrição para $f(P) = P$, i.e. para $f = id$. Quando o ponto branco acaba uma volta, o ponto vermelho acaba uma volta e portanto $deg(f) = 1$.
Escreva-se $P = (\cos(\theta), \sin(\theta))$ e tome-se como outro exemplo $f_n(P) = (\cos(n\theta), \sin(n\theta))$. Para $f_3$ temos então:
$deg(f_3) = 3$, porque o ponto vermelho dá três voltas enquanto o branco dá uma.
Para $f_{-1}$ temos $deg(f_{-1}) = -1$, tal como podemos ver na animação seguinte:
Quando o ponto branco dá uma volta no sentido positivo, o ponto vermelho dá uma volta no sentido negativo, daí o $-1$.
Claro que podemos pensar noutras funções contínuas, por exemplo $f(P) = -P$, a função que envia cada ponto para o seu ponto diametralmente oposto, o seu antípoda. Nesse caso, $deg(f) = 1$ já que:
Tendo presente a noção de grau de uma função contínua, afirmamos agora que o grau da função identidade $id: S^2 \to S^2$ é $1$ e que o grau da função antipodal $f: S^2 \to S^2$, $f: P \mapsto -P$ é $-1$ (note-se que em $S^1$ o grau da função antipodal era $1$!). Vamos provar o Teorema da Bola Cabeluda através de uma contradição.
O grau de uma função contínua $f$ é invariante sob homotopias, i.e. se $f$ for deformada continuamente o seu grau não se altera. Por outro lado, se existir um campo vetorial tangente que é sempre diferente de zero, para cada ponto $P$ em $S^2$ podemos definir $v_P$ como sendo esse vetor; com ele, definimos a homotopia $H: S^2\times [0, \pi] \to S^2$ de tal modo que $H(P, \theta)$ é o ponto que está a $\theta$ radianos de $P$, sobre o arco que vai de $P$ a $-P$ pela direção de $v_P$.
Deve ser claro que $H$ é contínua, que $H(P, 0) = P$ e que $H(P, \pi) = -P$, fazendo com que $H$ seja uma homotopia entre $id$ e $-id$, algo impossível! (porque são duas funções com graus diferentes). Tendo chegado a esta contradição concluímos que $v_P$ estava mal definido e que portanto havia um ponto $P$ onde a componente tangencial do campo vetorial era $0$!
O que acharam deste resultado? Quem diria que a matemática tem consequências tão engraçadas no mundo real..?
Em contextos menos rigorosos este teorema aparece escrito da seguinte forma:
Teorema (Bola Cabeluda I): se eu tiver uma bola cabeluda, independentemente do modo como a penteie haverá sempre um ponto onde os cabelos estão espetados no ar.
Em particular, nesta imagem em cima o cabelo está espetado tanto no topo como na parte de baixo da bola.
De um modo mais formal, o Teorema da Bola Cabeluda pode ser formulado como se segue:
Teorema (Bola Cabeluda II): qualquer campo vetorial contínuo sobre $S^2$ tem pelo menos um ponto onde a componente tangencial é $0$.
Com este teorema torna-se bastante fácil provar o nosso resultado!
Se pensarmos no vento sobre a superfície da Terra como um campo vetorial contínuo, o Teorema da Bola Cabeluda diz que tem de haver um ponto onde o vento não está a soprar para os lados!
Tudo o que nos falta agora é provar o teorema! Como podemos fazer isso? Vou usar uma prova da topologia algébrica porque eu acho que é uma prova relativamente intuitiva.
Para esta prova é preciso saber o que é o grau de uma função contínua de $S^n$ para $S^n$. Sem qualquer rigor, o grau de uma função contínua $f: S^n \to S^n$ pode ser visto como uma medida de quantas vezes $f(S^n)$ vai ser enrolado à volta de $S^n$.
Assumamos que estamos a trabalhar em $S^1$ (em $S^1$, o "grau" que se diz degree em inglês também tem o nome particular de winding number). Imaginem-se dois círculos lado a lado e, à medida que viajamos sobre o círculo da esquerda, em cima de um ponto $P$, vamos marcando no círculo da direita a imagem $f(P)$. O grau de $f$ vai ser o número de voltas completas que demos no círculo da direita quando acabarmos uma volta em cima do círculo da esquerda.
A animação em cima concretiza esta minha descrição para $f(P) = P$, i.e. para $f = id$. Quando o ponto branco acaba uma volta, o ponto vermelho acaba uma volta e portanto $deg(f) = 1$.
Escreva-se $P = (\cos(\theta), \sin(\theta))$ e tome-se como outro exemplo $f_n(P) = (\cos(n\theta), \sin(n\theta))$. Para $f_3$ temos então:
$deg(f_3) = 3$, porque o ponto vermelho dá três voltas enquanto o branco dá uma.
Para $f_{-1}$ temos $deg(f_{-1}) = -1$, tal como podemos ver na animação seguinte:
Quando o ponto branco dá uma volta no sentido positivo, o ponto vermelho dá uma volta no sentido negativo, daí o $-1$.
Claro que podemos pensar noutras funções contínuas, por exemplo $f(P) = -P$, a função que envia cada ponto para o seu ponto diametralmente oposto, o seu antípoda. Nesse caso, $deg(f) = 1$ já que:
Tendo presente a noção de grau de uma função contínua, afirmamos agora que o grau da função identidade $id: S^2 \to S^2$ é $1$ e que o grau da função antipodal $f: S^2 \to S^2$, $f: P \mapsto -P$ é $-1$ (note-se que em $S^1$ o grau da função antipodal era $1$!). Vamos provar o Teorema da Bola Cabeluda através de uma contradição.
O grau de uma função contínua $f$ é invariante sob homotopias, i.e. se $f$ for deformada continuamente o seu grau não se altera. Por outro lado, se existir um campo vetorial tangente que é sempre diferente de zero, para cada ponto $P$ em $S^2$ podemos definir $v_P$ como sendo esse vetor; com ele, definimos a homotopia $H: S^2\times [0, \pi] \to S^2$ de tal modo que $H(P, \theta)$ é o ponto que está a $\theta$ radianos de $P$, sobre o arco que vai de $P$ a $-P$ pela direção de $v_P$.
Deve ser claro que $H$ é contínua, que $H(P, 0) = P$ e que $H(P, \pi) = -P$, fazendo com que $H$ seja uma homotopia entre $id$ e $-id$, algo impossível! (porque são duas funções com graus diferentes). Tendo chegado a esta contradição concluímos que $v_P$ estava mal definido e que portanto havia um ponto $P$ onde a componente tangencial do campo vetorial era $0$!
O que acharam deste resultado? Quem diria que a matemática tem consequências tão engraçadas no mundo real..?
As animações foram feitas por mim, com código que se pode encontrar aqui.
- RGS